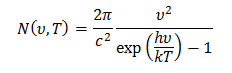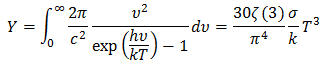About - Publications - Blog
The energy and photon flux emitted by the Sun and reaching Earth.
With some simple basic formulas, it is possible to estimate quite accurately the energy and number of photons emitted by the Sun and reaching Earth.
We first calculate the power of the Sun and the Earth. Then, we determine the corresponding number of photons (i.e. the photon flux). Finally, we calculate what fraction of the energy from the Sun reaches Earth.
What is the power of the Sun?
The Sun can be approximated by a black body. The Stefan–Boltzmann law describes the power radiated from a black body in terms of its temperature. The Stefan-Boltzmann law states that the total energy radiated per unit surface area of a black body across all wavelengths per unit time (also known as the black-body irradiance or emissive power) is:
P = 5.670400E-8. T^4
with T in K and P in W/m².
Depending on the temperature of the (surface of) the Sun (*), we obtain:
T = 5700 K => P = 5.99E+7 W/m²
T = 5778 K => P = 6.32E+7 W/m²
T = 5800 K => P = 6.42E+7 W/m²
T = 6000 K => P = 7.35E+7 W/m²
The radius of the Sun is 6.96E+8 m, resulting in a total surface area of 6.09E+18 m². Thus, the total power Ptot of the Sun is given by:
T = 5700 K => Ptot = 3.64E+26 W = 364 YW
T = 5778 K => Ptot = 3.85E+26 W = 385 YW
T = 5800 K => Ptot = 3.91E+26 W = 391 YW
T = 6000 K => Ptot = 4.47E+26 W = 447 YW
But how “big” is 385 YW? Click here to compare.
(*) depending on the source, the temperature of the surface of the Sun is different (it isn’t a constant by the way). Consensus seems to be 5778 K, but in a lot of textbooks, it is approximated by 5800 or even 6000 K. Notice the big difference in output energy (+16 %) if one approximates the temperature by 6000 K.
What is the power of the Earth?
Or more precisely, how much energy is radiating from the Earth per second if we approximate our planet by a black body?
The mean temperature of Earth is about T=287 K. Often, this temperature is approximated by 300 K.
With the Stefan–Boltzmann law, depending on the temperature of the (surface of) the Earth, we obtain:
T = 287 K => P = 385 W/m²
T = 300 K => P = 459 W/m²
The mean radius of the Earth is 6371 km, resulting in a total surface area of 5.10E+14 m². Thus, we obtain:
T = 287 K => Ptot = 1.96E+17 W = 196 PW
T = 300 K => Ptot = 2.34E+17 W = 234 PW
But how “big” is 196 PW? Click here to compare.
By the way, the Earth is not a black body, so this is only a rough approximation.
How many photons are leaving the Sun?
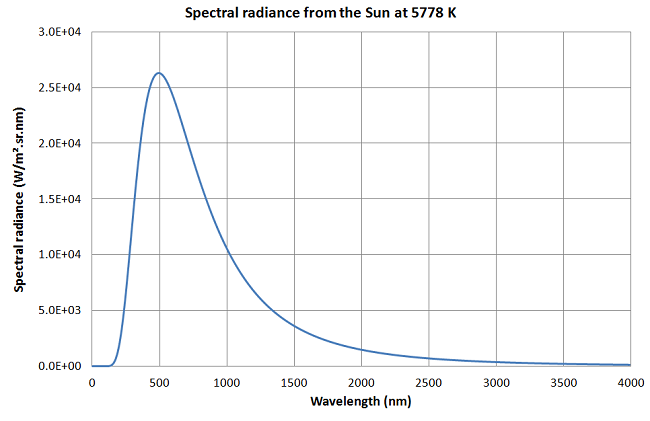
The spectral photon radiance (number of photons leaving per unit of frequency) of a black body is given by Planck's spectrum:
This formula gives the number of photons N per certain frequency v (in Hz) leaving a black body on absolute temperature T (in K), per m² and per s. The unit of N is here: 1/(m².s.Hz)
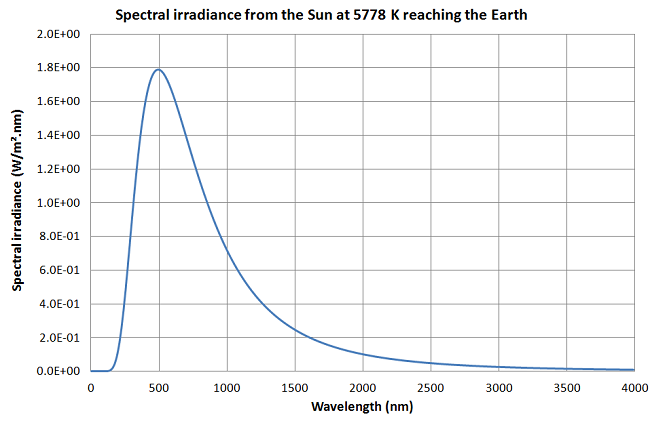
Integrating over the full spectrum, results into:
with ζ the zeta function of Riemann, k the constant of Boltzmann, and
![]()
the Stefan-Boltzmann constant. The unit of Y is 1/(m².s)
The zeta function of 3 (also called Apéry's constant), with (too) many decimals is:
ζ(3) = 1.202056903159594
Depending on the our approximation of the Sun, we obtain
T = 5700 K => Y = 2.82E+26 /m².s
T = 5778 K => Y = 2.93E+26 /m².s
T = 5800 K => Y = 2.97E+26 /m².s
T = 6000 K => Y = 3.28E+26 /m².s
How many photons from the Sun are reaching Earth?
If we want to know how many photons from the Sun reach Earth, we have to take into account the geometrical factor (view factor), given by (radius Sun / distance Sun-Earth)². Note that this is an average value, because of the eccentricity of Earth's orbit.
Multiplying with the geometrical factor = 2.18E-5 results into the number of photons from the Sun reaching Earth (T = temperature of the Sun):
T = 5700 K => Y = 6.14E+21 /m².s
T = 5778 K => Y = 6.39E+21 /m².s
T = 5800 K => Y = 6.47E+21 /m².s
T = 6000 K => Y = 7.16E+21 /m².s
The average energy of a photon from the Sun reaching the Earth is:
T = 5700 K => average = (1292 W/m²) / (6.14E+21 / m².s)
= 2.10 E-19 J (= 1.31 eV)
T = 5778 K => average = (1364 W/m²) / (6.39E+21 / m².s)
= 2.13 E-19 J (= 1.33 eV)
T = 5800 K => average = (1385 W/m²) / (6.47E+21 / m².s)
= 2.14 E-19 J (= 1.34 eV)
T = 6000 K => average = (1586 W/m²) / (7.16E+21 / m².s)
= 2.22 E-19 J (= 1.38 eV)
How many photons are leaving the Earth?
Analogous, one can calculate the number of photons leaving Earth, if one approximates Earth by a black body with temperature T.
T = 287 K => Y = 3.59E+22 /m².s
T = 300 K => Y = 4.11E+22 /m².s
The average energy of a photon leaving the Earth is:
T = 287 K => average = (385 W/m²) / (3.59E+22 / m².s)
= 1.07 E-20 J (= 0.067 eV)
T = 300 K => average = (459 W/m²) / (4.11E+22 / m².s)
= 1.12 E-20 J (= 0.070 eV)
How much energy from the Sun is reaching the Earth?
We calculate the spectral radiance B_lambda(T) i.e. the energy emitted per
second, per m², per steradian, per m wavelength: W / (m² . sr. m)
![]()
For a wavelength of 502 nm (where the maximum occurs), the spectral radiance
is 2.63E+13 W/(m².sr.m).
Per nanometer wavelength we obtain 2.63E+4 W/(m².sr.nm)
The following graph shows the spectral radiance in W/m².sr.m:

Usually, the spectral radiance is expressed per nm:
If we want to get rid of the steradian, we have to multiply by pi by reason of Lambert's cosine law. This results, for our example of 502 nm, in 8.27E+4 W/(m².nm)
We then obtain the spectral irradiance in W/m².nm:
Because of the inverse
square law, the energy arriving at Earth will be (for 502 nm):
8.27E+4 W/(m².nm) * (radius Sun / distance Sun-Earth)² =
8.27E+4 W/(m².nm) * (6.96342E+8 m / 149.60E+9 m )² = 1.79 W/(m².nm)
Plotting this spectral irradiance P from the Sun, reaching the Earth, in W/m².nm gives following graph:
Depending on the approximation of the temperature T of
the (surface of) the Sun, we obtain:
T = 5700 K => P(502 nm) = 1.67 W/(m².nm)
T = 5778 K => P(502 nm) = 1.79 W/(m².nm)
T = 5800 K => P(502 nm) = 1.83 W/(m².nm)
T = 6000 K => P(502 nm) = 2.16 W/(m².nm)
Integrated over the entire spectrum, we obtain the solar constant Z:
T = 5700 K => Z = 1294.4 W/m²
T = 5778 K => Z = 1366.7 W/m²
T = 5800 K => Z = 1387.7 W/m²
T = 6000 K => Z = 1589.2 W/m²
The solar constant is not a constant (who invents those stupid names?). Consensus value is about 1361 W/m², corresponding (with our (very good) approximation of a black body) with a temperature of the surface of the Sun of 5772 K.
To be complete, we plot the spectral radiance reaching the Earth, in W/m².sr.nm:
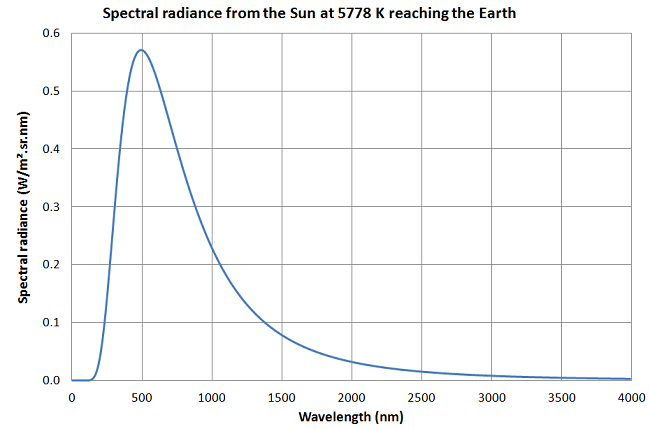
How many photons reach the Earth every second?
The spectral radiance from the Sun reaching the Earth in W/m².sr.nm is given by:

.
The spectral radiance peaks at 502 nm, corresponding to 0.570 W/m².sr.nm.
The energy E of each photon at a given wavelength λ is given by:
E = (h.c) / λ
with h Planck's constant and c the speed of light. For example, the energy of a photon with a wavelenght of 502 nm is 3.96E-19 J = 396 zJ.
Dividing the spectral radiance by the energy at each wavelength gives us the spectral photon flux.
For example, for 502 nm:
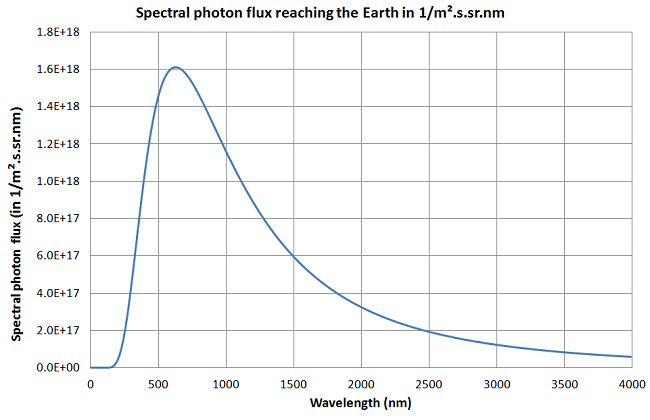
(0.570 W/m².sr.nm) / 396 zJ = 1.44 E+18 / m².s.sr.nm
or multiplication
by pi gives us 4.52E+18 / m².s.nm
The spectral photon flux in 1/m².s.sr.nm:
The spectral photon flux in 1/m².s.nm:
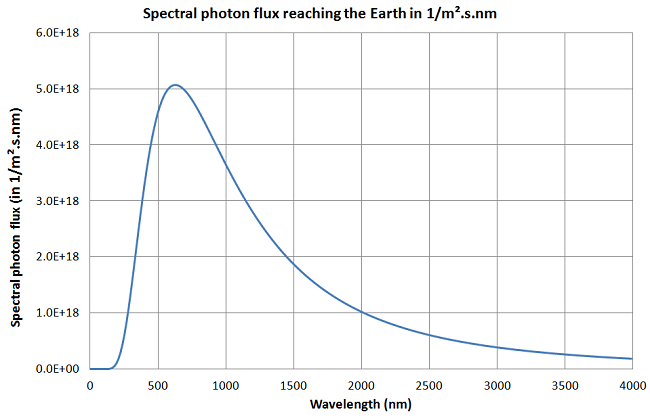
(Please note that the maximum is not anymore located at 502 nm, because, the higher the wavelength, the lower the corresponding energy.)
The total photon flux can be calculated by integrating over the entire spectrum. We obtain a total photon flux of 6.35E+21 / m².s. In other words, every second 6.35E+21 photons are reaching one square meter of the Earth.
The mean radius of the Earth is 6731 km. This means the light of the Sun falls on a surface of about 1.42E+14 m² (quick approximation). Conclusion: every second, about 9E+35 photons reach the Earth. Give or take a few of course.
(Just a reminder: these calculations assume that the Sun is an ideal black body. This is an approximation. For a more detailed spectrum, search for "A.M.0 spectrum").
What fraction of the solar energy reaches Earth?
We calculated how much energy is leaving the Sun. It is a lot: 385 YW (= 3.85E+26 W).
We also calculated how much energy of the Sun in reaching Earth (at the top of our atmosphere). It's the solar constant 1364 W/m².
The mean radius of the Earth is 6371 km. The Earth is (very well approximated by) a sphere. The total surface area equals 4*pi*6371² km² = 510064041 km² = 5.10E+14 m².
The Sun only shines on one half of the planet at once, thus the power reaching
the Earth from the Sun is:
1364 W/m² * 2.55E+14 m² = 3.48E+17 W
But is this correct? The radiation from the Sun is not perpendicular on the
entire surface. If we consider the circle diameter surface of the Earth, we
get a more accurate approximation:
1364 W/m² * (pi*6371² km²) = 1.74E+17 W
What fraction of the solar energy then reaches Earth?
1.74E+17 W / 3.85E+26 W = 4.5E-10 = 0.000000045 %
Let us check if this number is correct, using solid angle of the Earth on the
surface of the Sun, or easier explained:
(the circle diameter surface of the Earth)
divided by
(the total surface area of a sphere with radius the distance between the Sun
and the Earth)
= (pi*6371² km²) / (4*pi*(1.5E+11)² m²) = 4.5E-10 = 0.000000045
%