Single variable expressions for the efficiency of a reciprocal power transfer system

Summary

Any power transfer system can be represented by a two port network, characterized by its impedance matrix. In the past, it was already shown that it is possible to express the efficiency of the power transfer as function of the elements of this impedance matrix. In this work, we now demonstrate that the efficiency can be expressed as function of a single parameter. Moreover, this single parameter, which we call the extended kQ factor, has a physical meaning. We apply our results on wireless power transfer systems.

Reference

Ben Minnaert and Nobby Stevens. Single variable expressions for the efficiency of a reciprocal power transfer system. International Journal of Circuit Theory and Applications, 45 (10), (2017). 1418-1430.
Paper: [pdf]

Introduction

A general reciprocal power transfer system can be represented as a two-port network with at the first port, a voltage source, and at the second port, a passive load (Figure 1). Power is transferred from the voltage source to the load.

Note that we do not specify the circuitry used for the power transfer. We consider the circuit as a black box, characterized by its impedance matrix Z.

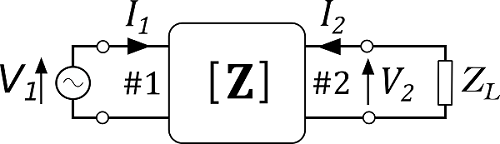
Figure 1: A general reciprocal power transfer system, represented as a two-port network with a voltage source and a passive load at the terminals.

Efficiency expressions

The efficiency of the power transfer is defined as the ratio between the active output power delivered to the load, and the active input power supplied by the voltage source. If we consider the source and the impedance matrix fixed, a certain value of the load is necessary to maximize the efficiency of the power transfer.

We have demonstrated that this maximum efficiency can be expressed as function of a single figure of merit, i.e., the extended kQ factor alpha. The maximum efficiency eta_max is given by:

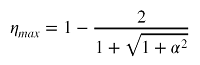
Instead of maximizing the efficiency of the power transfer, it is also possible to maximize the amount of power transfer. Another value of the load is then necessary. The efficiency of the power transfer for the maximum power configuration is again expressable as function of a single parameter, the extended kQ factor alpha. The efficiency eta_power is:

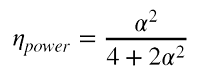
Figure 2 shows the relation between the efficiency and the extended kQ factor for three configurations: the maximum efficiency and power transfer configuration, described above, and the conjugate image configuration (described in the paper referenced above).

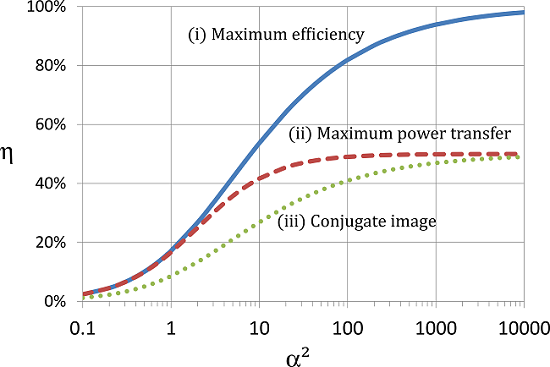
Figure 2: The efficiency of any reciproqual power transfer network as function of its extended kQ factor squared.

Inductive wireless power transfer

The reason why this single parameter alpha is called the extended kQ factor can be found within the example of inductive wireless power transfer. Consider two coupled coils as given in Figure 3.

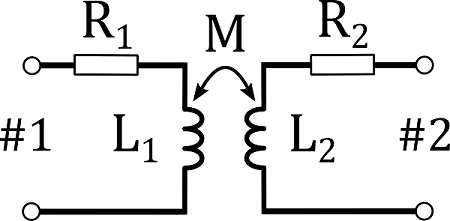
Figure 3: An inductive wireless link.

Calculation of the extended kQ factor alpha results in:

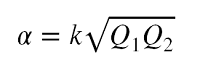
which, for L1=L2 and R1=R2 results in alpha=kQ. In other words, the single parameter that describes the efficiency of any reciproqual power transfer system equals the kQ factor for inductive wireless power transfer, thus the name 'extended kQ factor' for other power transfer systems.