Conjugate Image Theory Applied on Capacitive Wireless Power Transfer

Summary

In order to obtain a maximum power transfer efficiency, an optimal compensation network must be designed at the input and output ports of a capacitive wireless power transfer system. In this work, the conjugate image theory is applied to determine this optimal network as function of the characteristics of the capacitive wireless link. We also compare inductive and capacitive wireless power transfer and apply the conjugate image theory for mixed coupling. We demonstrate that the results for inductive and capacitive coupling can be deduced from this mixed configuration.

References

Ben Minnaert and Nobby Stevens. Conjugate Image Theory Applied on Capacitive Wireless Power Transfer. Energies, 10 (1), (2017) art.nr. 46
Paper: [pdf]

Ben Minnaert and Nobby Stevens. Conjugate image theory for non-symmetric inductive, capacitive and mixed coupling. Wireless Power Transfer Conference (WPTC), 2017 IEEE. Taipei, Taiwan, 10-12 May 2017.
Paper: [pdf]

Overview

Let's start with an example. We consider a simple inductive wireless power transfer system: power is transferred from a voltage source VS (at a certain operating frequency) to a load RL by a pair of coupled inductors (Fig. 1).

Fig. 1: A basic inductive wireless power transfer circuit.

A question we might ask is: how can we maximize the efficiency of the power transfer? (note: this is not the same as maximizing the amount of power transfer).

The answer is choosing optimal values for the load, and for the resonating capacitances (e.g., Cs1 and Cp2 on Fig. 2). These optimal values can easily be calculated, e.g., by circuit network theory.

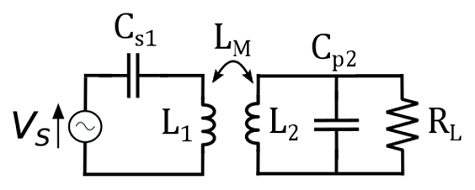
Fig. 2: Optimized inductive wireless power transfer circuit.

Congratulations! You just applied the conjugate image theory. This theory allows the easy calculation of the optimal values for maximazing the power transfer efficiency of any power transfer system.

Fig. 3 shows a general power transfer system, characterized by its impedance matrix Z. The conjugate image theory calculates the optimal values for Z1, Z2 and the load ZL in order to maximize the power transfer efficiency from the source to the load.

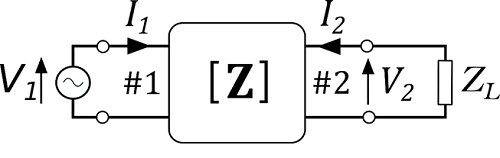
Fig. 3: any power transfer system can be represented by a two-port network, characterized by its impedance matrix Z.

In this work, the conjugate image theory is applied for the first time on capacitive and mixed wireless power transfer systems. Interesting similarities can be found between inductive and capacitive wirelss power transfer, as they can be deduced from a mixed configuration.